Try the Free Math Solver or Scroll down to Tutorials!

Enter expression, e.g. (x^2-y^2)/(x-y)

Enter expression, e.g. x^2+5x+6

Enter expression, e.g. (x+1)^3

Enter a set of expressions, e.g. ab^2,a^2b

Enter equation to solve, e.g. 2x+3=4

Enter equation to graph, e.g. y=3x^2-1

Depdendent Variable

Number of equations to solve:

Equ. #1:

Equ. #2:

Equ. #3:

Equ. #4:

Equ. #5:

Equ. #6:

Equ. #7:

Equ. #8:

Equ. #9:

Solve for:

Enter inequality to solve, e.g. 2x+3>4

Enter inequality to graph, e.g. y<3x^2-1

Dependent Variable

Number of inequalities to solve:

Ineq. #1:

Ineq. #2:

Ineq. #3:

Ineq. #4:

Ineq. #5:

Ineq. #6:

Ineq. #7:

Ineq. #8:

Ineq. #9:

Solve for:

Math solver on your site

Please use this form if you would like
to have this math solver on your website,
free of charge.

Name:

Email:

Your Website:

Msg:

Write Linear Equations in Standard Form

Goal • Use point-slope form to write equations in
standard form.

Example 1 Write Linear Equations in Standard Form

Write two equations in standard form that are
equivalent to 4x + 2y = 12.

Solution
To write one equivalent equation, multiply each side
by 0.5 .
2x + y = 6
To write another equivalent equation, multiply each side
by 2 .
8x + 4y = 24

√Guided Practice Complete the following exercises.
1. Write two equations in standard form that are
equivalent to 6x - 4y = 6.
3x - 2y = 3; 12x - 8y = 12

2. Write two equations in standard form that are
equivalent to -12x + 6y = 29.
-4x + 2y = 23; -24x + 12y = -18

Example 2 Write an equation from a graph

Write an equation in standard form of the line shown.

Solution
Step 1 Calculate the slope.

Step 2 Write an equation in point-slope form.
Use the point (2, 4).

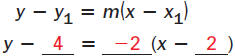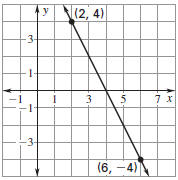
Write point-slope form.
Substitute 4 for y₁,
-2 for m, and 2
for x₁.

Step 3 Rewrite the equation in standard form.

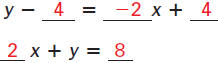Simplify.
Collect variable terms on one
side, constants on the other.

√Guided Practice Complete the following exercise.

3. Write an equation in standard form of the line
through (3, -1) and (2, -4).

y - 3x = -10

Example 3 Write an equation of a line

Write an equation of the specified line.

a. Line A

b. Line B

Solution

a. Line A is a vertical line, so
all points on the line have an
x-coordinate of 3. An
equation of the line is x = 3.

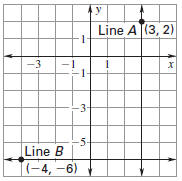b. Line B is a horizontal line, so all points on the line
have a y-coordinate of -6. An equation of the line is
y = -6.

Example 4 Complete an equation in standard form

Find the unknown coefficient in the equation of the line
shown. Write the completed equation.

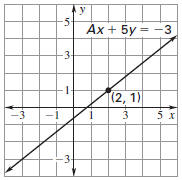
Solution

Step 1 Find the value of A. Substitute the coordinates of
the given point for x and y in the equation. Solve
for A.

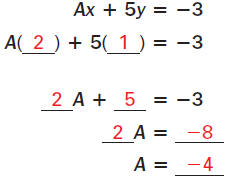Write equation.

Substitute 2 for x and
1 for y.

Simplify.
Subtract 5 from each side.
Divide by 2 .

Step 2 Complete the equation.
-4 x + 5y 5 -3 Substitute -4 for A.

√Guided Practice Complete the following exercise.

4. Write equations of the horizontal and vertical lines
that pass through (-10, 5).
Horizontal: y = 5; Vertical: x = -10

5. Find the missing coefficient in the equation of
the line that passes through (-2, 2). Write the
completed equation.
6x + By = 4
B = 8; 6x + 8y = 4